White's strategy of not allowing black to place a block of "6-squares".
How can Black avoid White's intentions?
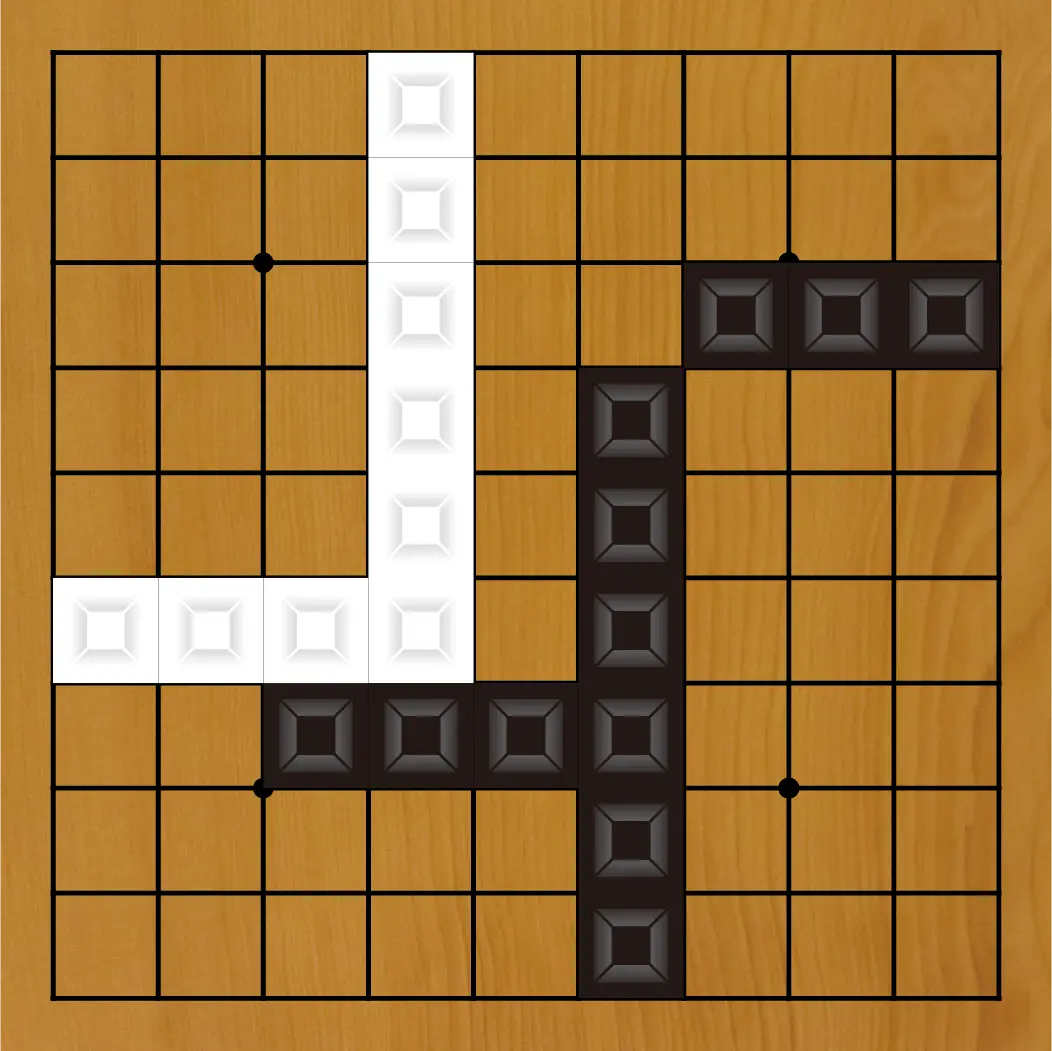
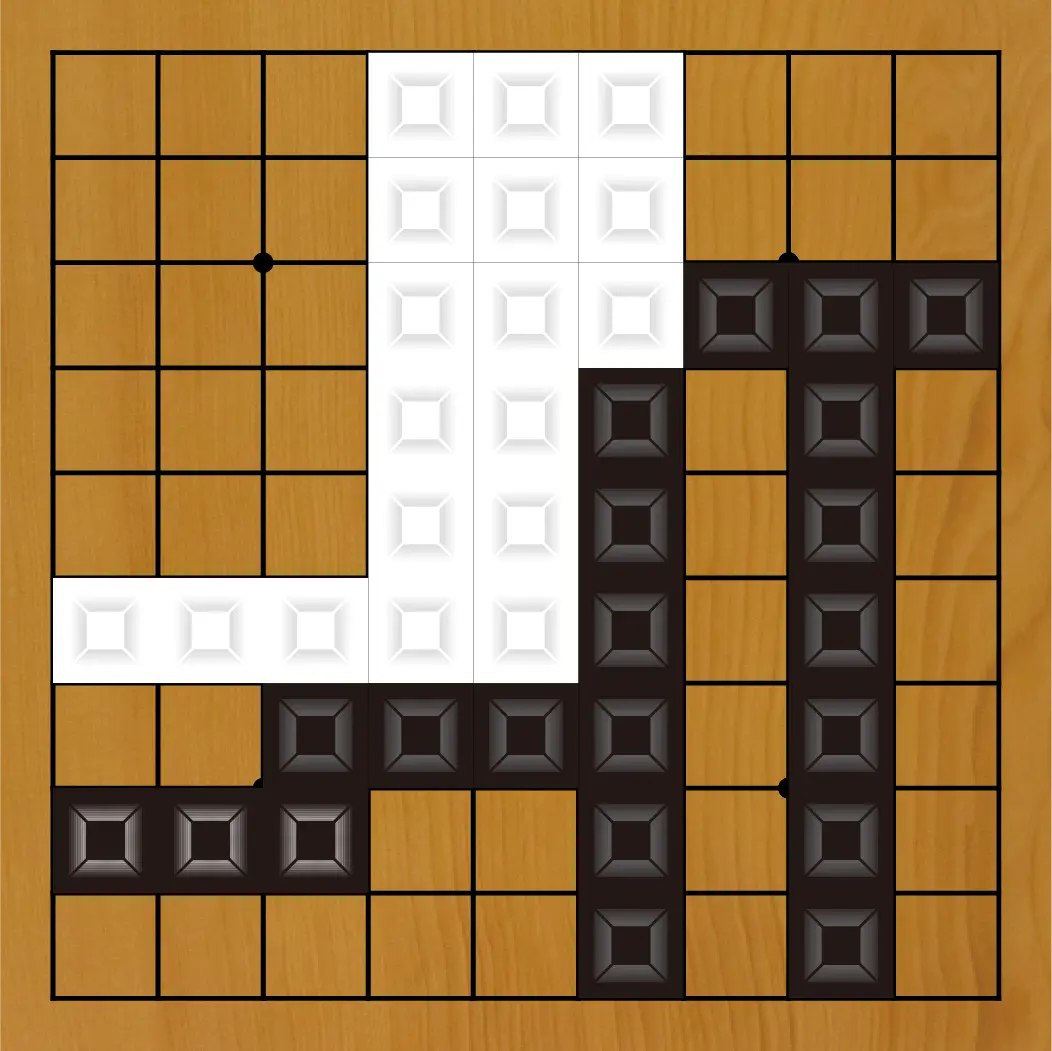
White has placed a block of "3-squares" in the Black
territory.
The intention of this move is to prevent Black from placing a
block of "6-squares".
If Black fails to place a block of "6-squares", the block of
"6-squares" will remain in the block case until the end.
Black should not be provoked by White and should deal with the
situation calmly.
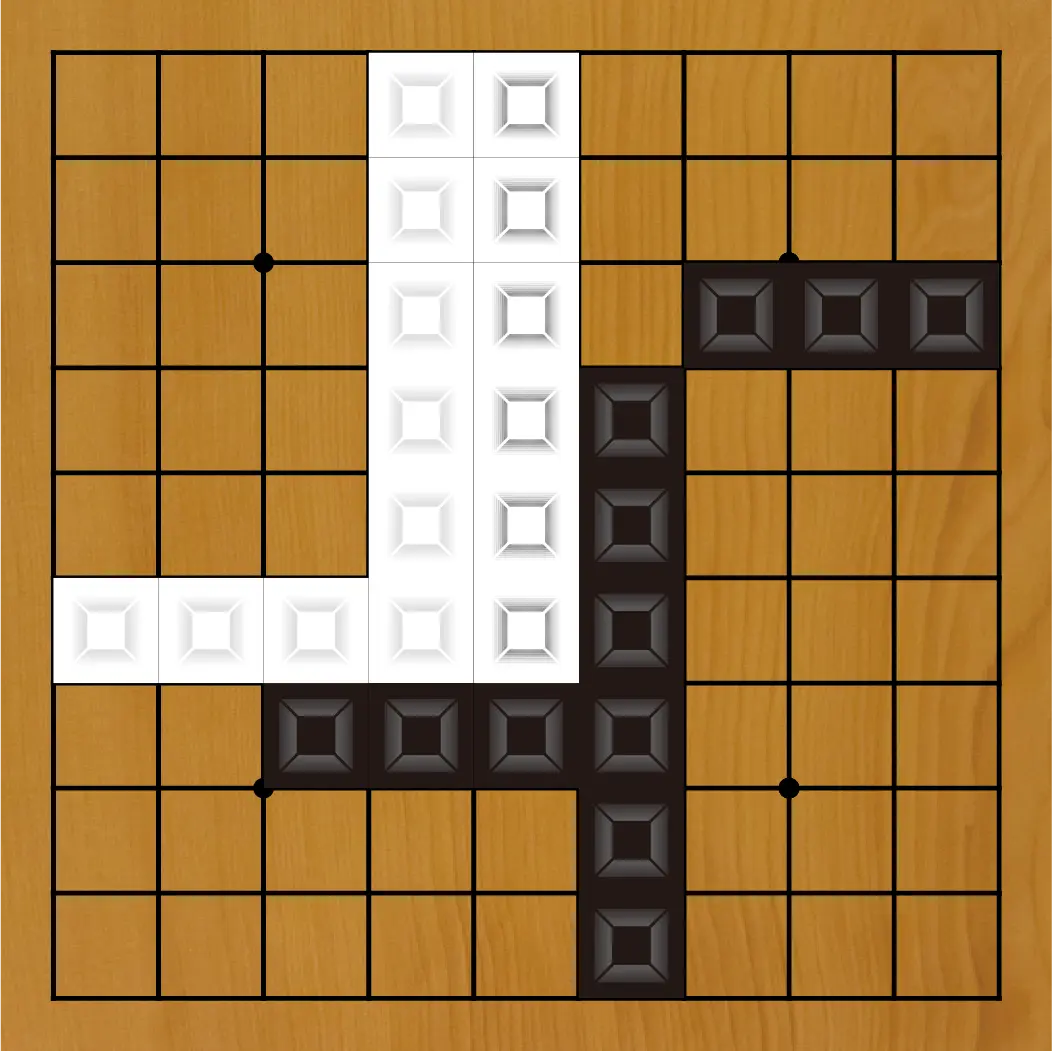
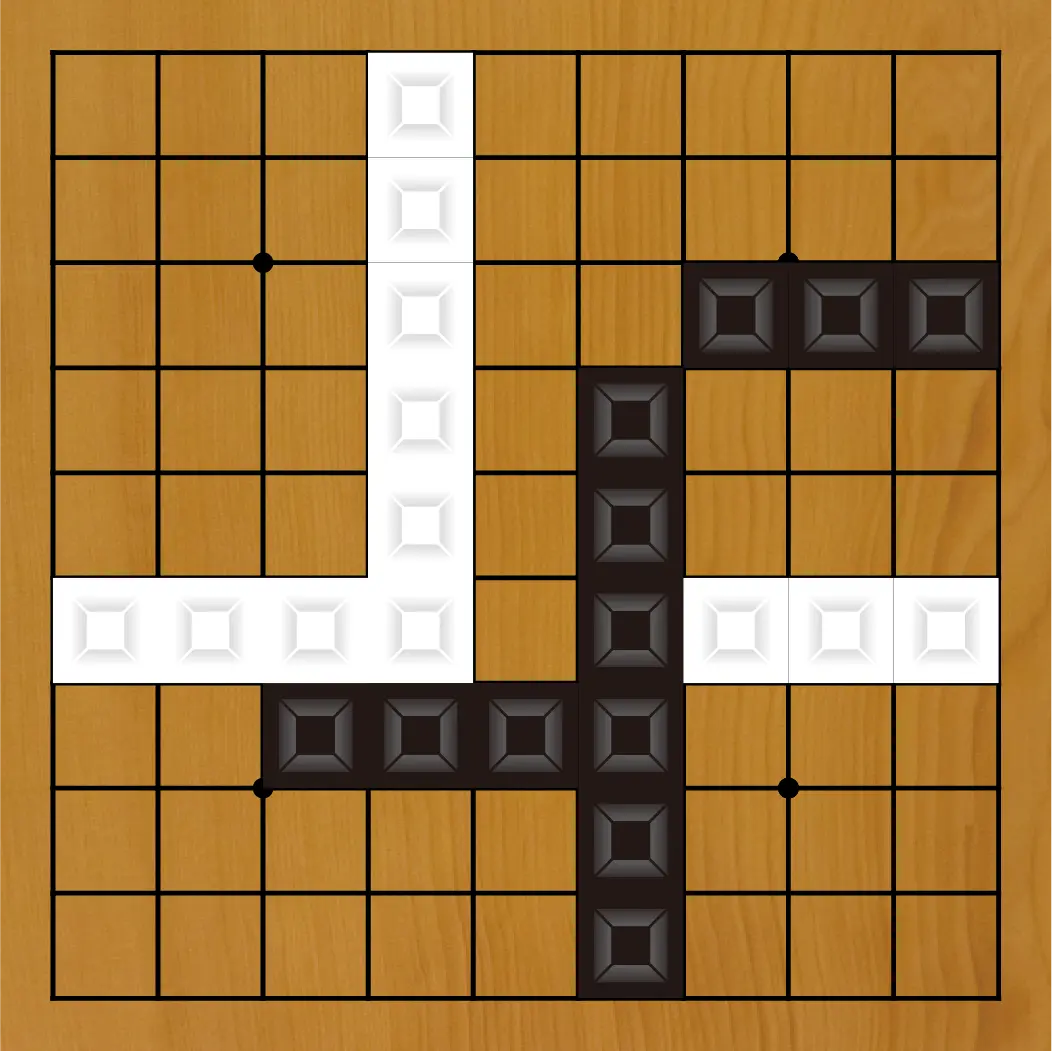
Question 5-1
Where should Black place his blocks to avoid White's intentions?

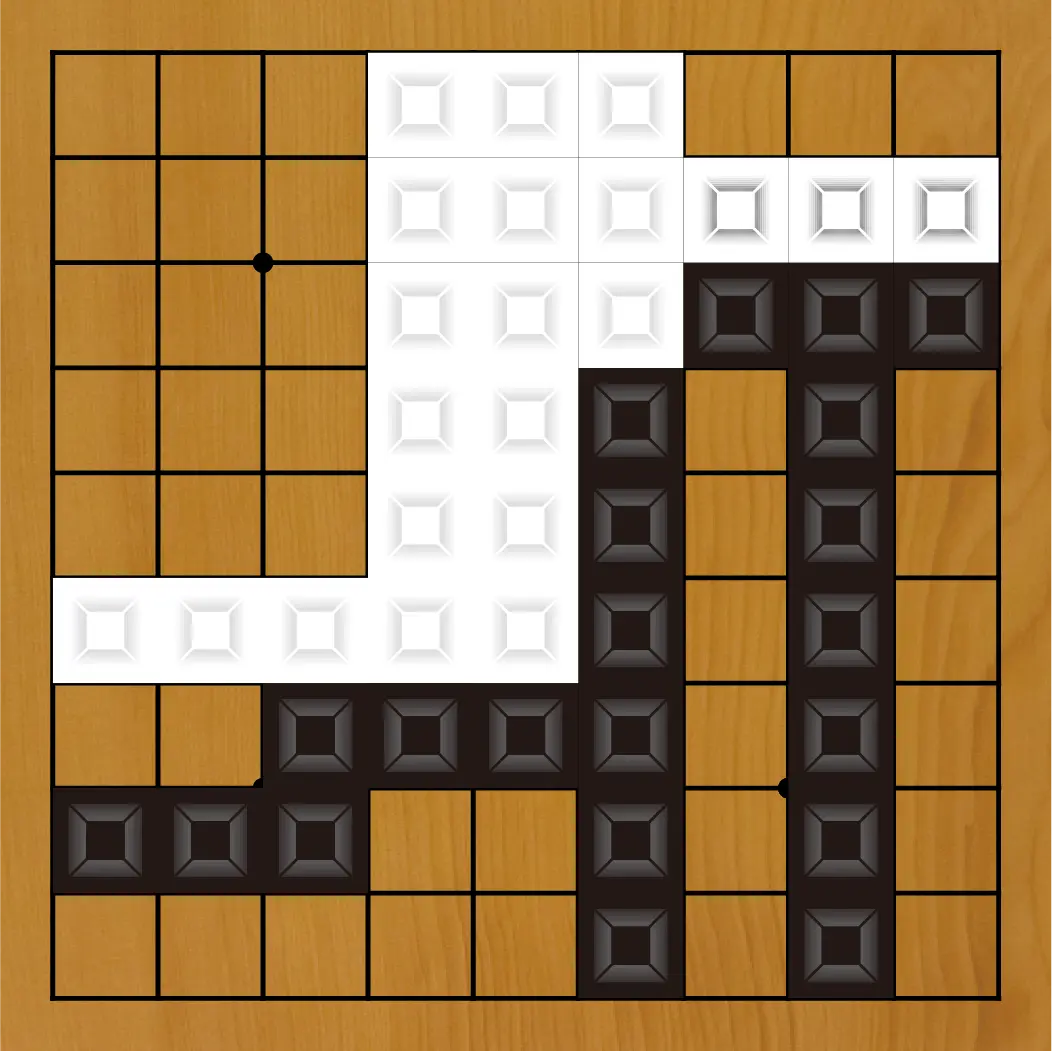
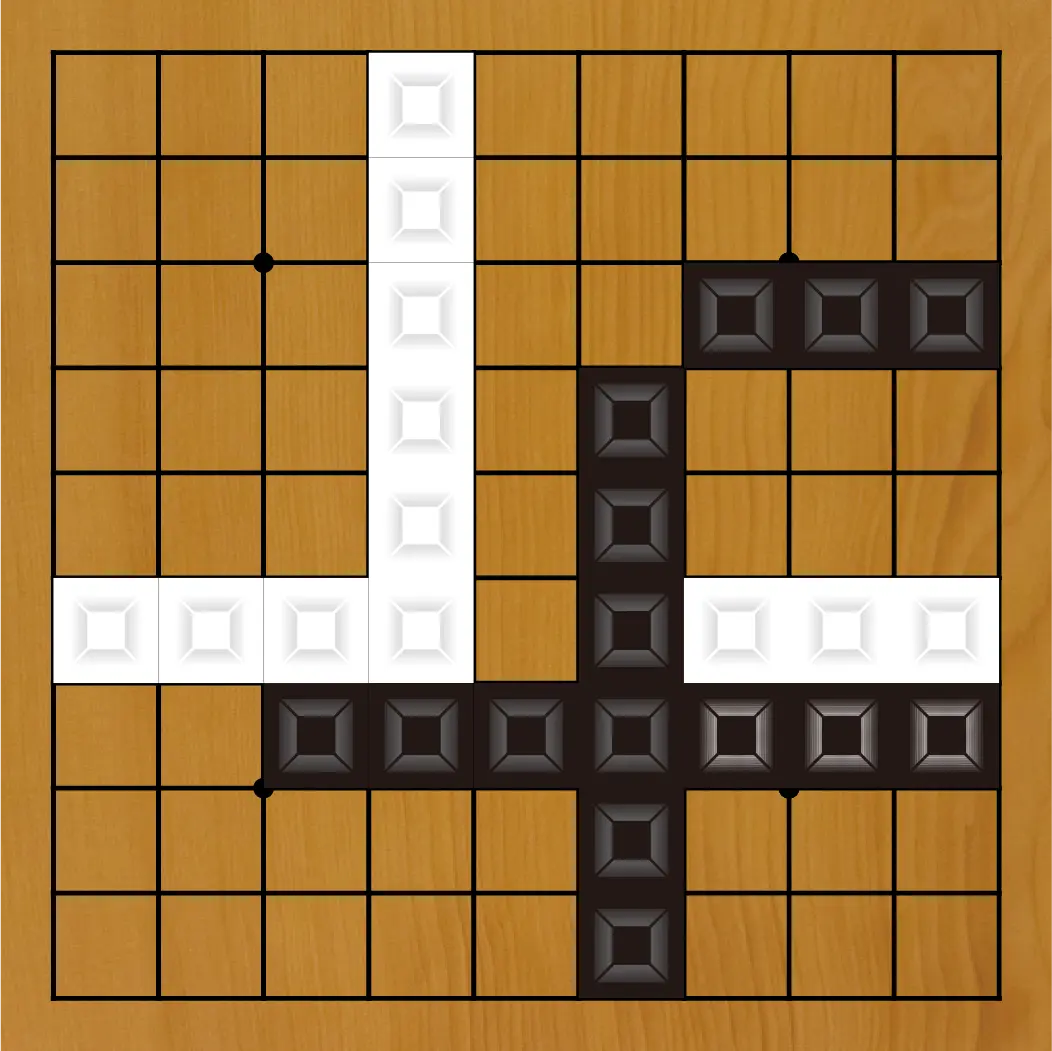
Failure
5-1 F
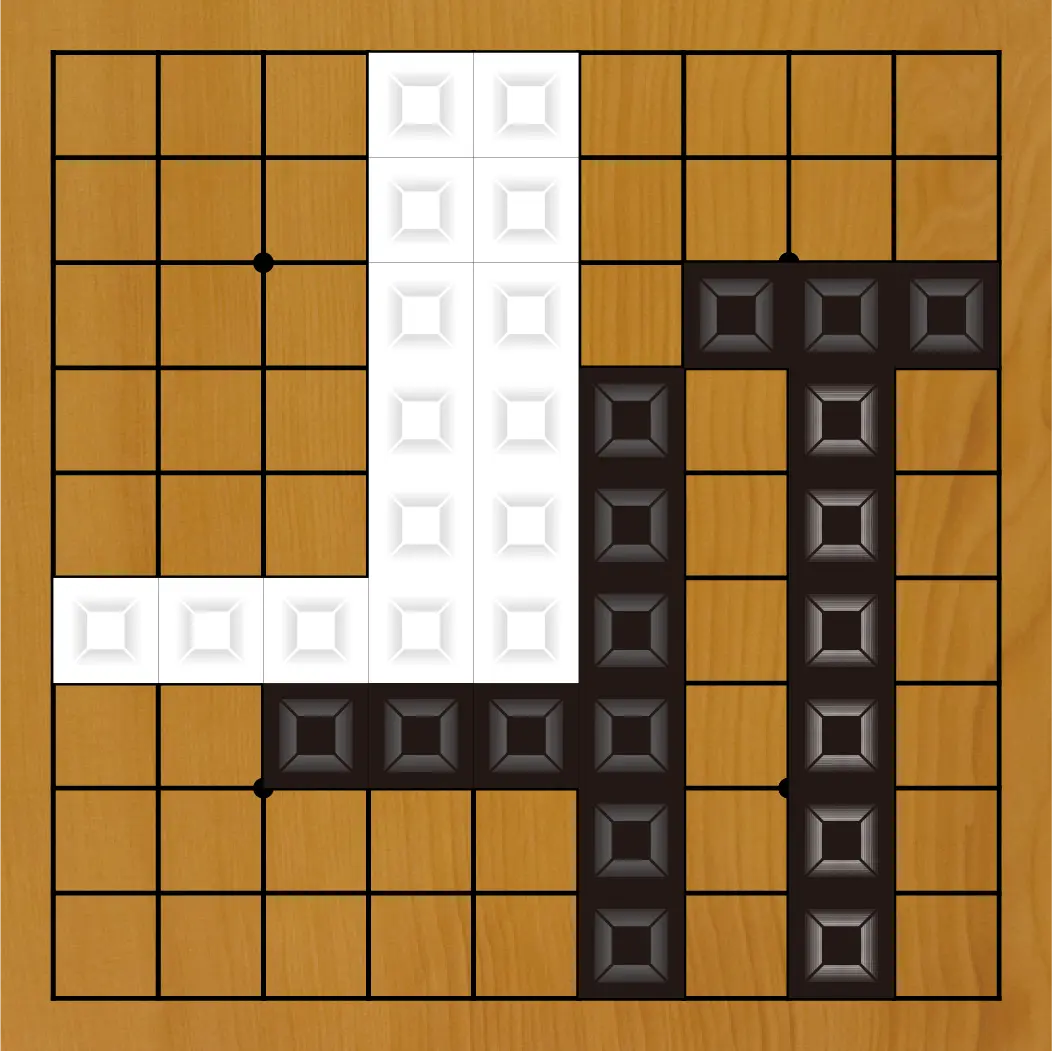
Black should not aim at white block of "3-squares" placed in the Black's territory.
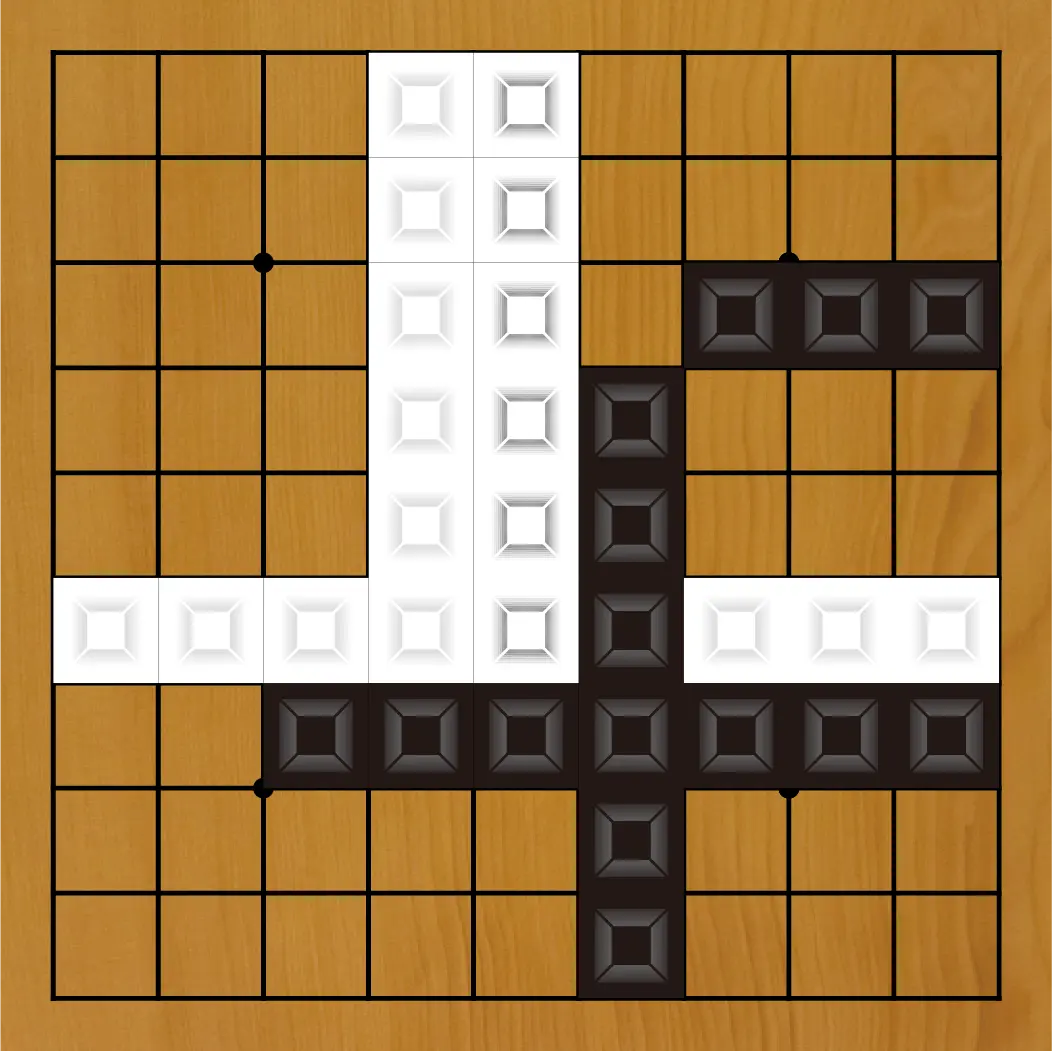
White has placed a block of "6-squares" and Black can no longer place a block of "6-squares" anywhere on the board.
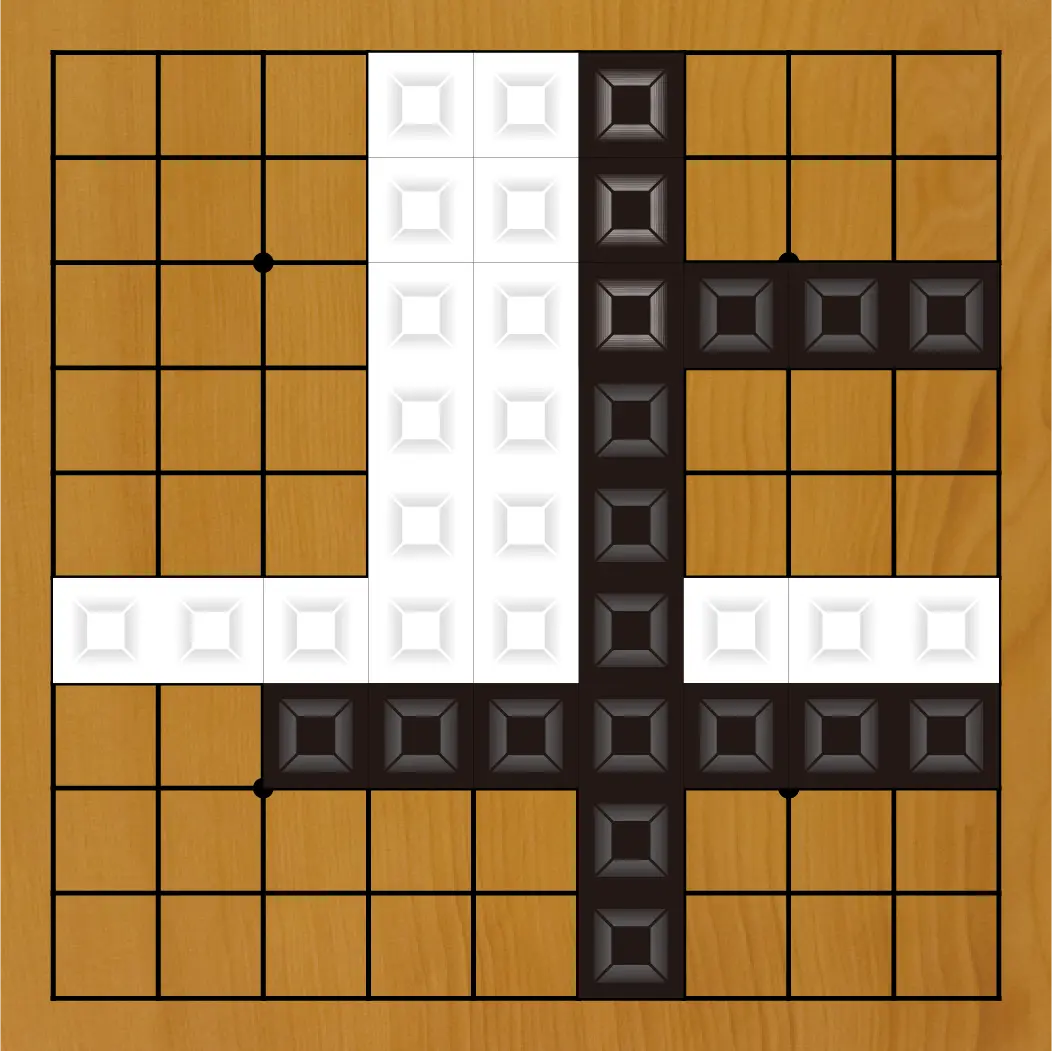
Even if Black enclosed his territory with a block of "3 squares",
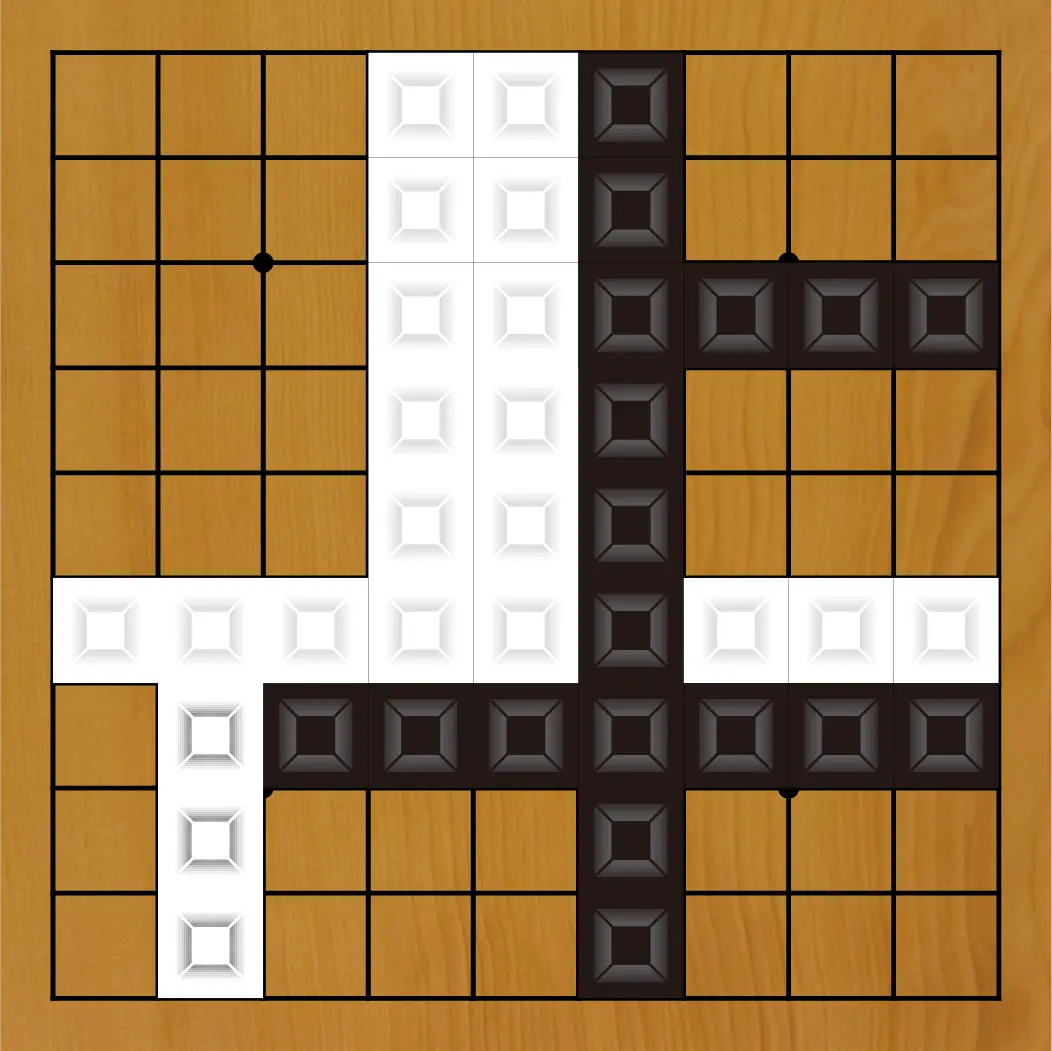
If White encloses his territory with a block of "3-squares", White wins.
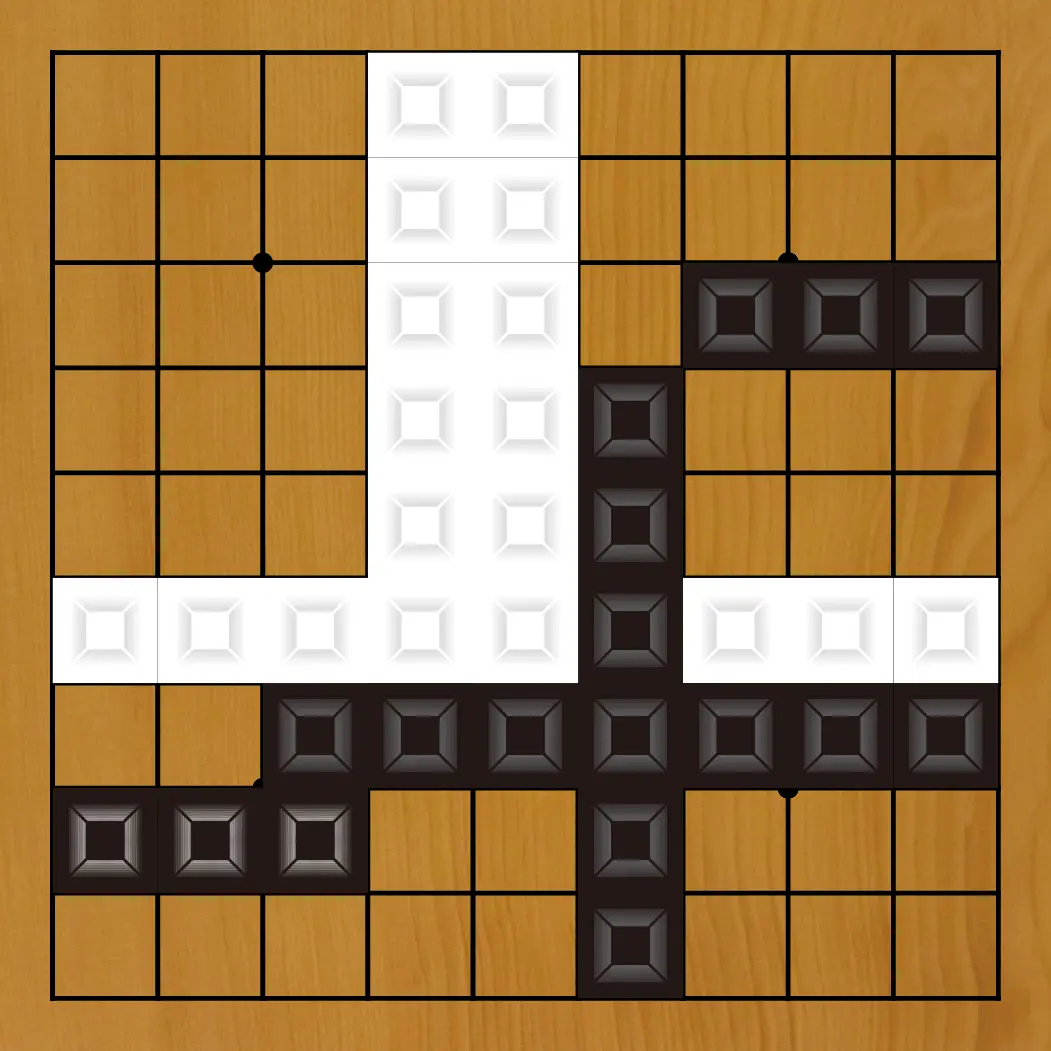
Even if Black enclosed his territory with a block of "3 squares",
White wins by placing a block of "3-squares" to reduce Black's territory.

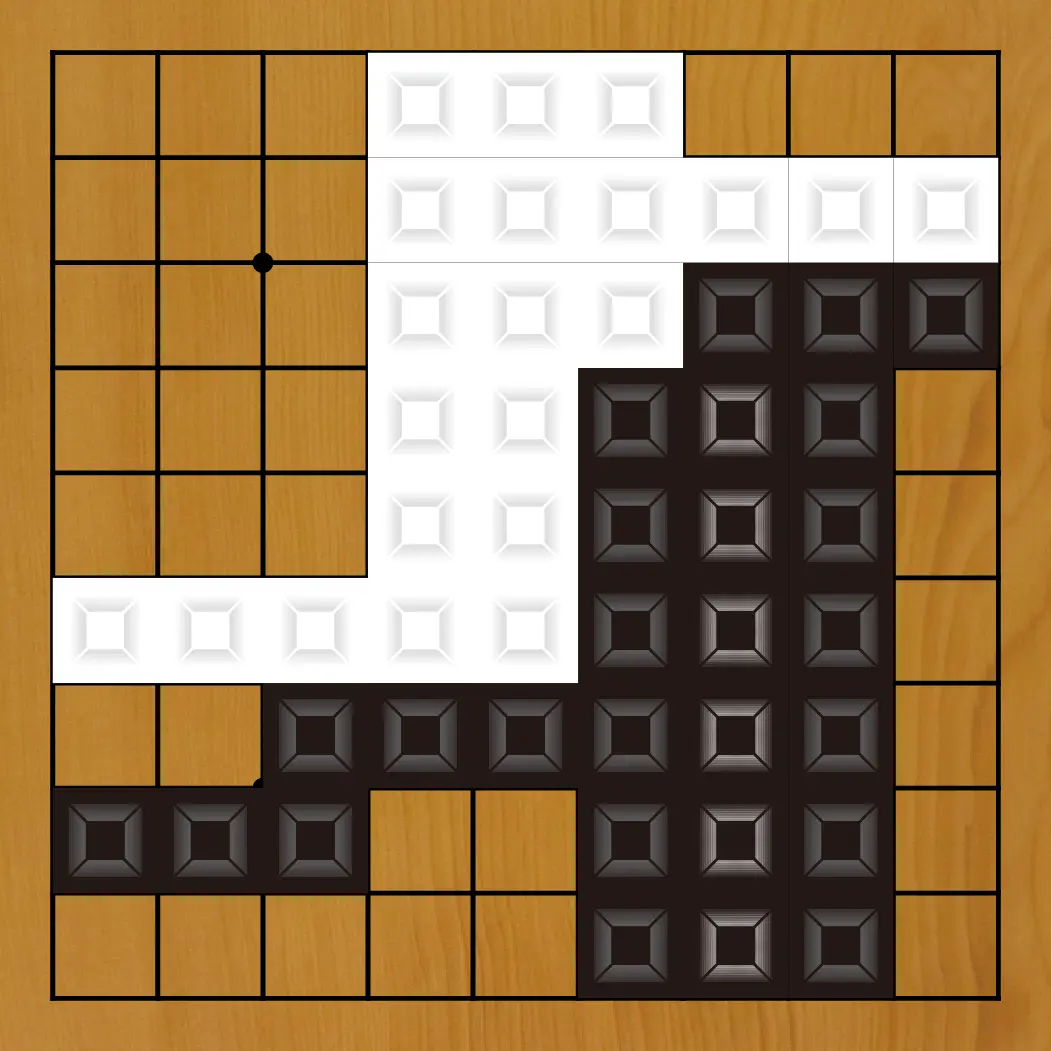
Success
5-1 S
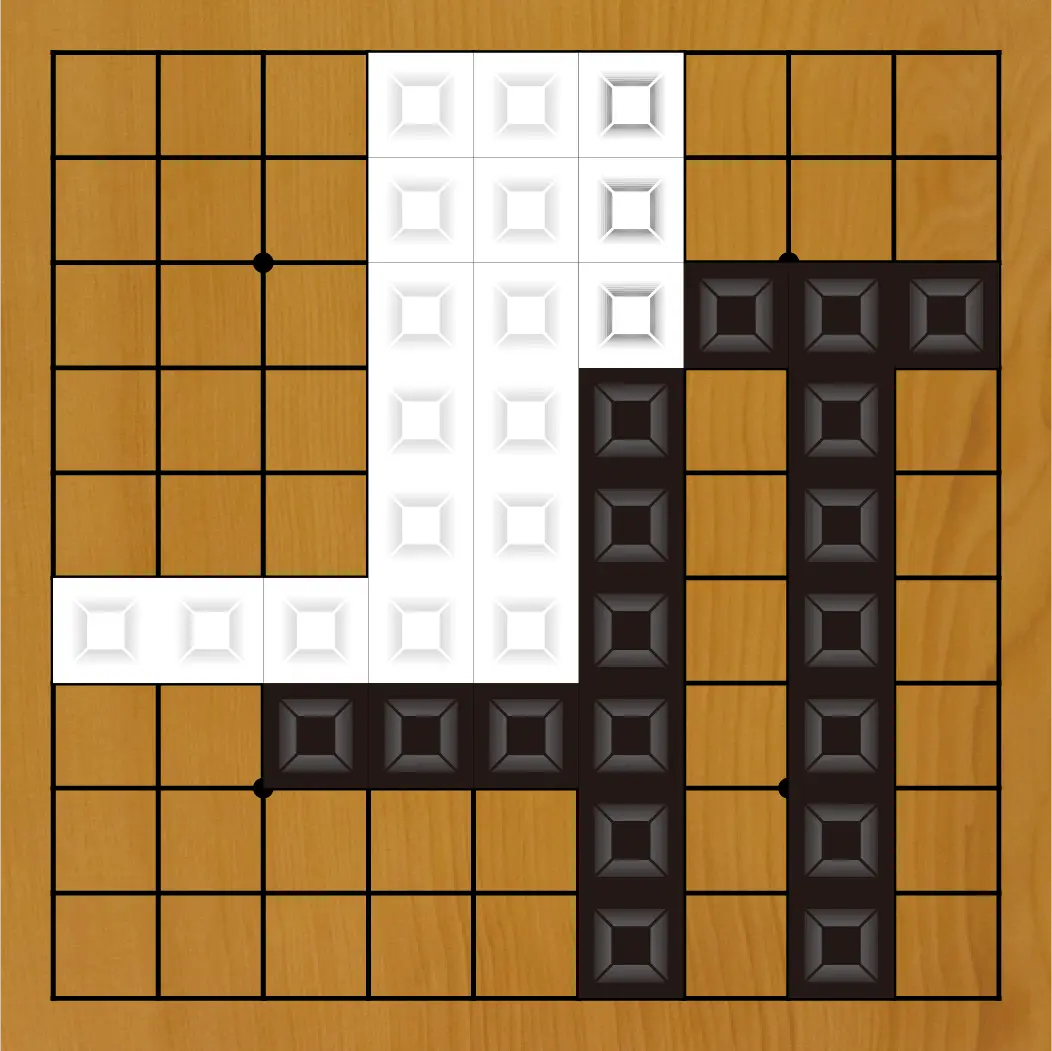
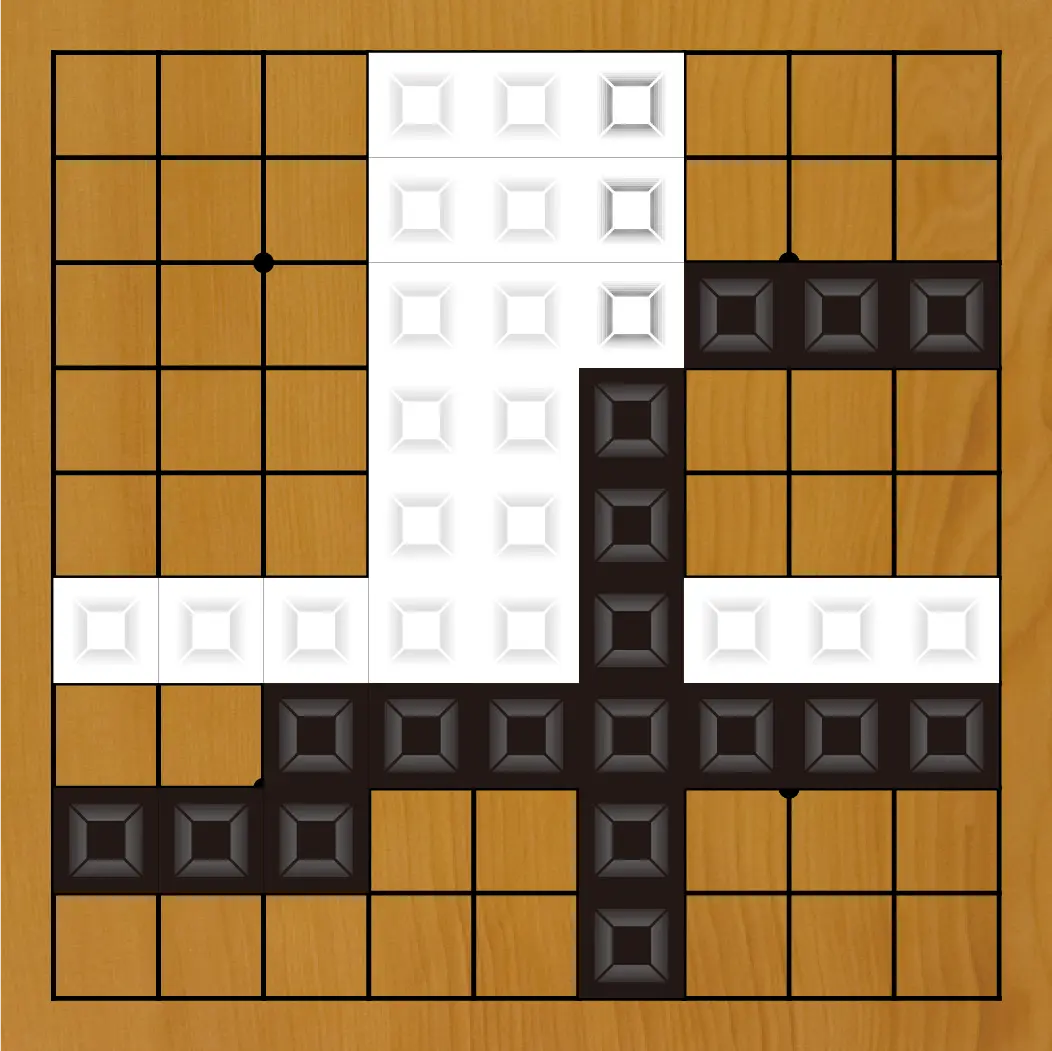
Black's smart strategy is to place a block of "6-squares" to extend his territory.
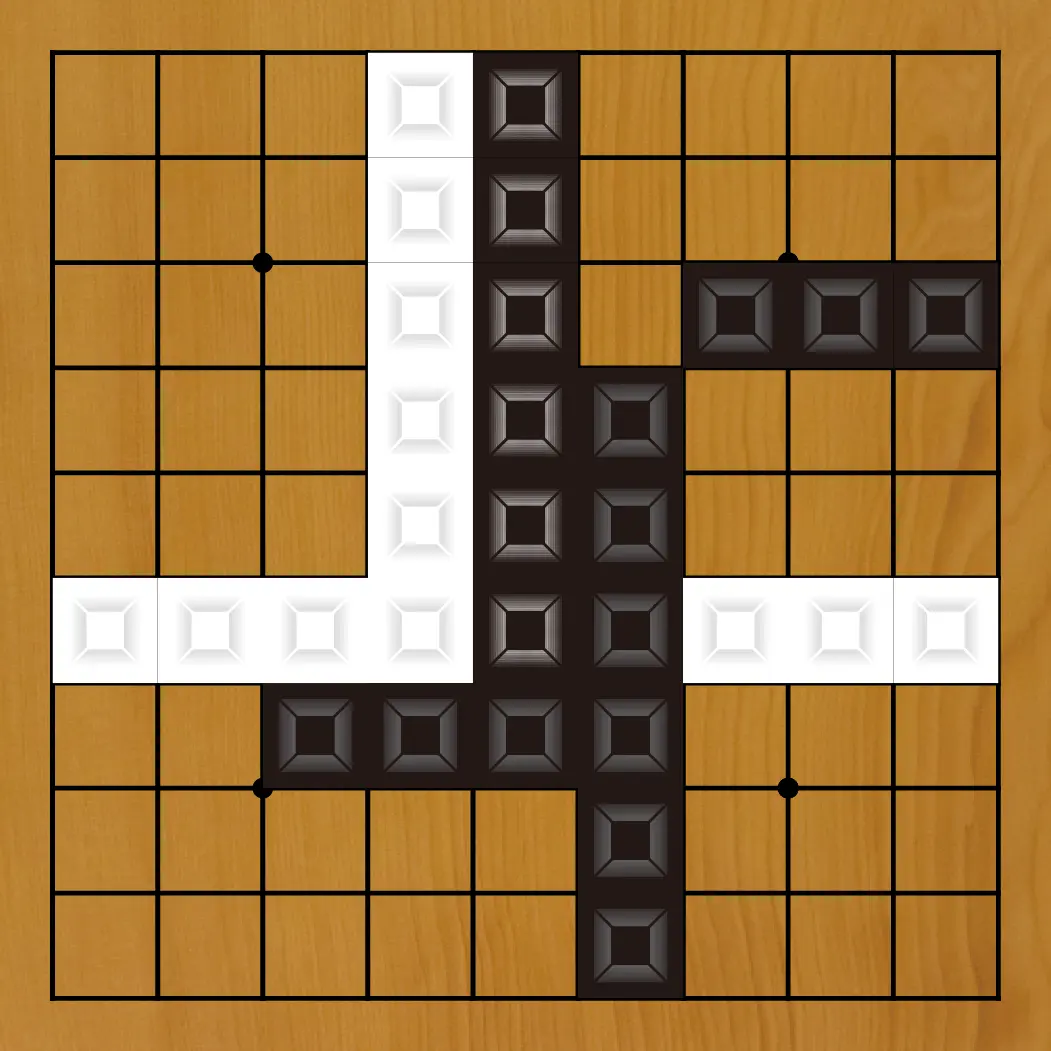
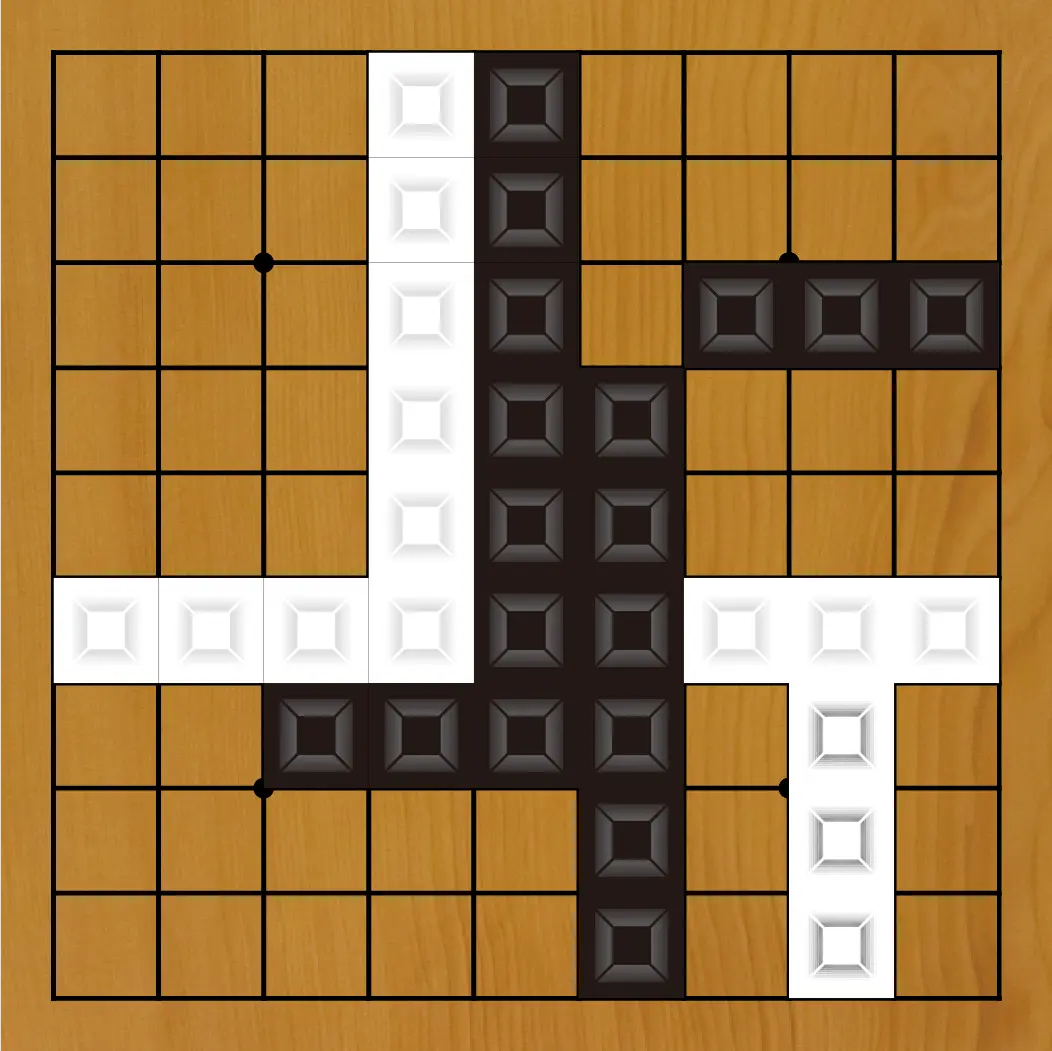
White placed a block of "3-squares" to create a "boo" and he was
able to create his territory in the corner.
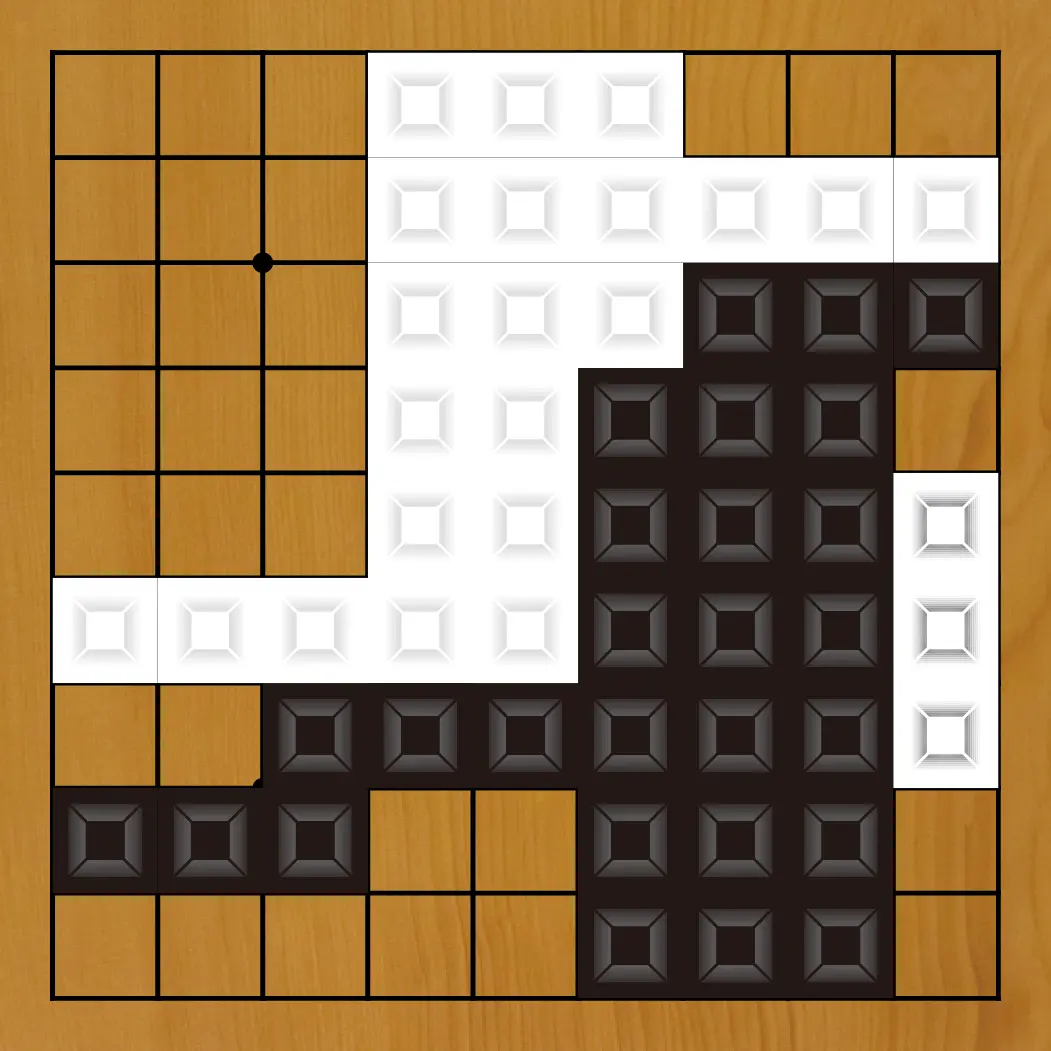
White seems to have succeeded in reducing Black's territory in
the corner, but
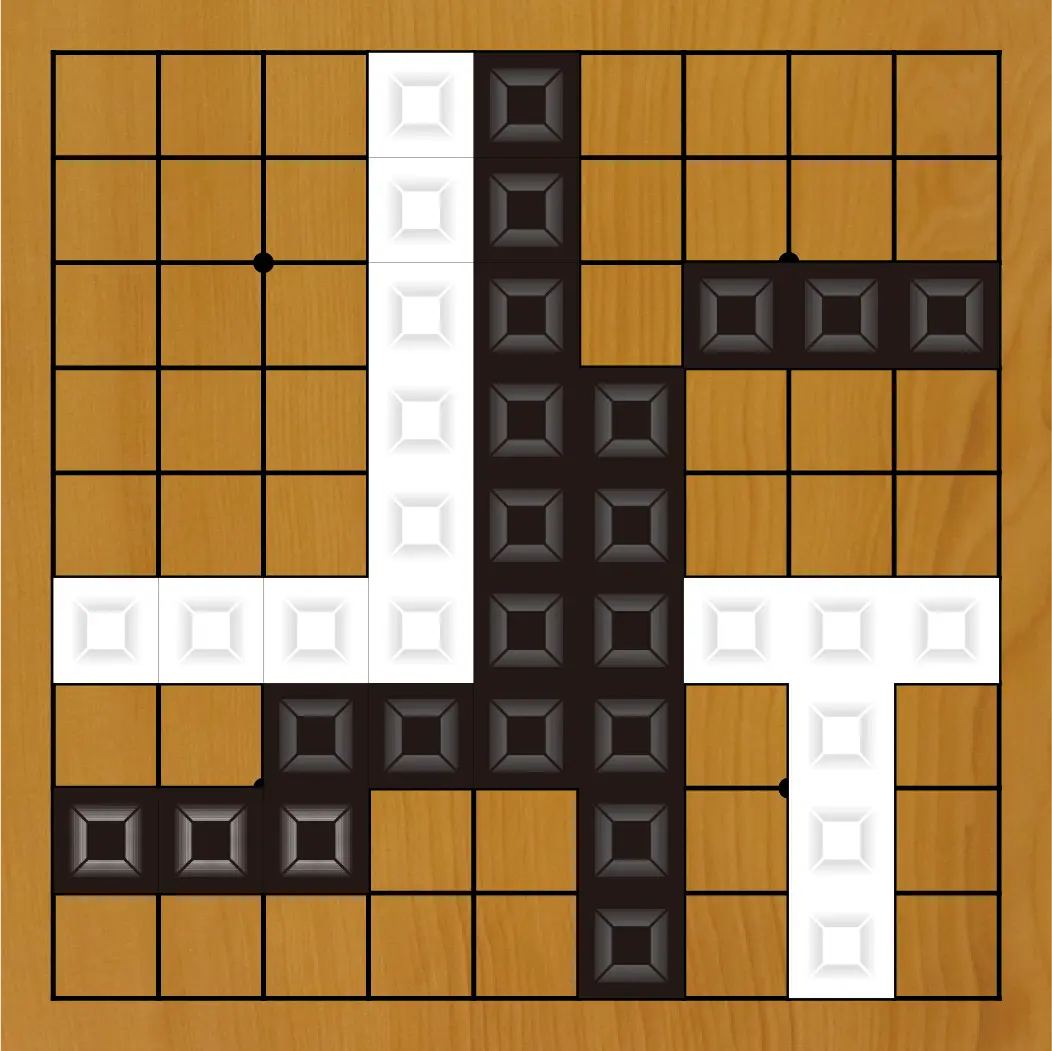
Black encloses his territory by placing a block of
"3-squares".
Black's territory is more than White's territory, so Black wins.